Further Mathematics JAMB, WAEC, NECO AND NABTEB Official Past Questions

(a) A(-1, 2), B(3, 5) and C(4, 8) are the vertices of triangle ABC. Forces whose magnitudes are 5N and \(3\sqrt{10}\)N act along \(\overrightarrow{AB}\) and \(\overrightarrow{CB}\) respectively. Find the direction of the resultant of the forces.

(b) A particle starts from rest and moves in a straight line. It attains a velocity of 20 m/s after covering a distance of 8 metres. Calculate : 

(i) its acceleration ; (ii) the time it will take to cover a distance of 40 metres.

(a) Given that \(x = 3i – j, y = 2i + kj\) and the cosine of the angle between x and y is \(\frac{\sqrt{5}}{5}\), find the values of the constant k.

(b) In the quadrilateral ABCD, 

 \(\overrightarrow{AB} = \begin{pmatrix} -5 \\ -1 \end{pmatrix}, \overrightarrow{AC} = \begin{pmatrix} -6 \\ -9 \end{pmatrix}\)

and \(\overrightarrow{BD} = \begin{pmatrix} 4 \\ -7 \end{pmatrix}\). Show whether or not ABCD is a parallelogram.

The probabilities that Kofi, Kwasi and Ama will pass a certain examination are \(\frac{9}{10}, \frac{4}{5}\) and x respectively. If the probability that only one of them will pass the examination is \(\frac{9}{50}\), find the :

(a) value of x ; 

(b) probability that at least one of them will pass the examination.

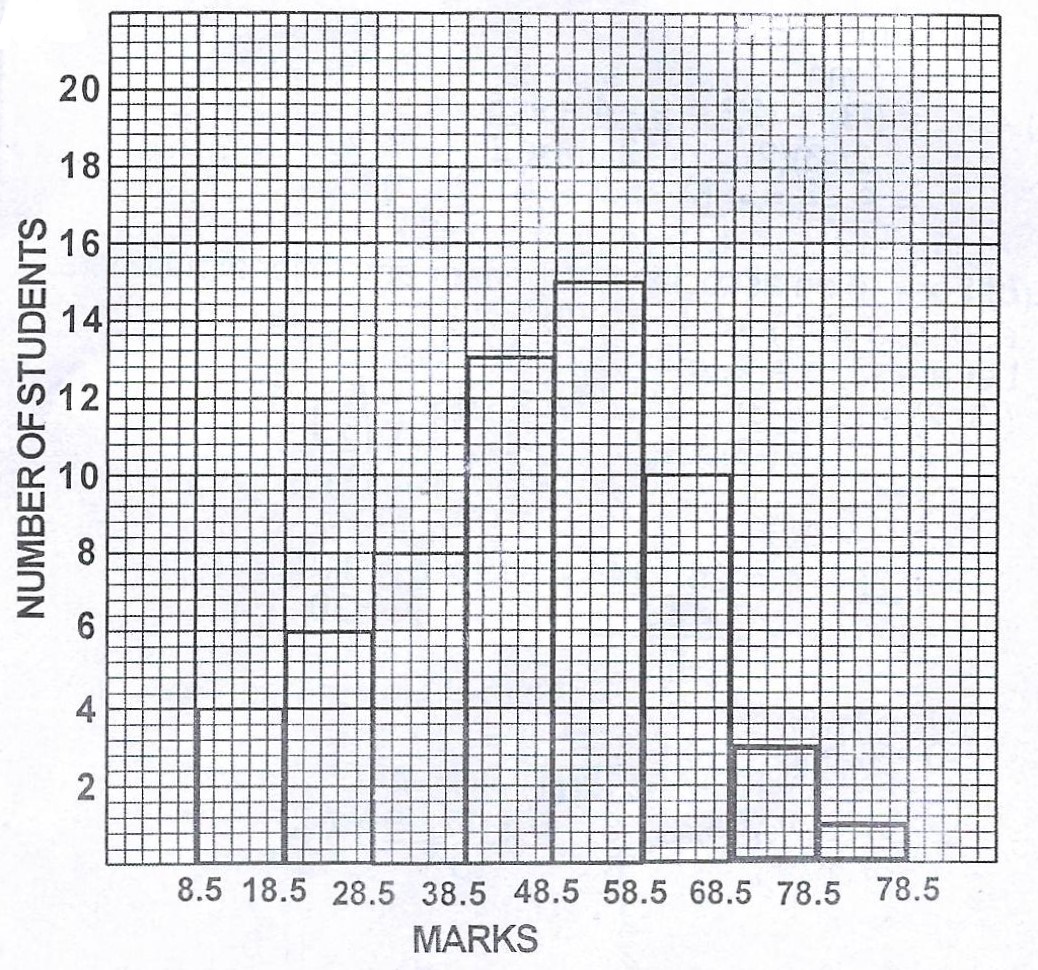
The histogram above represents the scores of some candidates in an examination. 

(a) Using the histogram, construct a frequency distribution table indicating clearly the class intervals ;

(b) Draw a cumulative frequency curve of the distribution and use it to estimate the :

(i) median ; (ii) quartile deviation.

(a) If \(f(x) = \frac{x – 3}{2x – 1} , x \neq \frac{1}{2}\) and \(g(x) = \frac{x – 1}{x + 1}, x \neq -1\), fing \(g \circ f\).

(b)(i) Sketch the curve \(y = 9x – x^{3}\) ; (ii) Calculate the total area bounded by the x- axis and the curve \(y = 9x – x^{3}\).

(a) Express \(\frac{5 + \sqrt{2}}{3 – \sqrt{2}} – \frac{5 – \sqrt{2}}{3 + \sqrt{2}}\) in the form \(a + b\sqrt{2}\).

(b) Solve the following equations simultaneously using the determinant method.

\(3x – y – z = -2\)

\(x + 5y + 2z = 5 \)

\(2x + 3y + z = 0\)

(a) Differentiate \((x – 3)(x^{2} + 5)\) with respect to x.

(b) If \((x + 1)^{2}\) is a factor of \(f(x) = x^{3} + ax^{2} + bx + 3\), where a and b are constants, find the :

(i) values of a and b ; (ii) zeros of f(x).

 Find the direction of the resultant of the forces in the diagram.

The table shows the distribution of the lengths of 20 iron rods measured in metres :

Using an assumed mean of 1.45, calculate the mean of the distribution.

A committee of 3 is formed from a panel of 5 men and 3 women. Find the :

(a) number of ways of forming the committee ;

(b) probability that at least one woman is on the committee.

The position vectors of P, Q and R are \(11i + j, 5i + \frac{13}{3}j\) and \(2i + 6j\) respectively. 

(a) Show that P, Q and R lie on a straight line.

(b) Find the ratio of \(|\overrightarrow{PQ}| : |\overrightarrow{QR}|\)

Find the gradient of \(xy^{2} + x^{2} y = 4xy\) at the point (1, 3).

If \(\alpha\) and \(\beta\) are the roots of \(3x^{2} + 5x + 1 = 0\), evaluate \(27(\alpha^{3} + \beta^{3})\).

A binary operation \(\ast\) is defined on the set of rational numbers by \(m \ast n = \frac{m^{2} – n^{2}}{2mn}, m \neq 0 ; n \neq 0\).

(a) Find \(-3 \ast 2\).

(b) Show whether or not \(\ast\) is associative.

Solve \((\log_{2} m)^{2} – \log_{2} m^{3} = 10\).

Express (14N, 240°) as a column vector.

Evaluate \(\frac{\tan 120° + \tan 30°}{\tan 120° – \tan 60°}\)

Find \(\lim\limits_{x \to 3} (\frac{x^{3} + x^{2} – 12x}{x^{2} – 9})\)

Find the distance between the points (2, 5) and (5, 9).

A ball is thrown vertically upwards with a velocity of 15\(ms^{-1}\). Calculate the maximum height reached. \([g = 10ms^{-2}]\)

If the points (-1, t -1), (t, t – 3) and (t – 6, 3) lie on the same straight line, find the values of t.