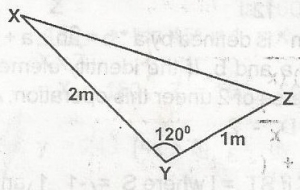
find the length XZ in the triangle
-
\(\sqrt{7m}\)
- \(\sqrt{6m}\)
- \(\sqrt{5m}\)
- \(\sqrt{3m}\)
The correct answer is: A
Explanation
xz2 = xy2 + yx2 - 2(xy) (yz) cos 120o= 22 + 12 - 2(2) (1) cos 1202
= 4 + 1 - 4x - cos 60 = 5 - 4x - \(\frac{1}{2}\)
5 + 2 = 7
xz = \(\sqrt{7}\)m