The sum of the ages of Musa and Lawal is 28 years. After sharing a certain sum of money in the ratio of their ages, Musa gets N600 and Lawal N800. How old is Lawal?
- A. 14 years
- B. 20 years
- C. 12 years
- D. 16 years
If y = (1 + x)2, find \(\frac{dy}{dx}\)
- A. x - 1
- B. 2 + 2x
- C. 1 + 2x
- D. 2x - 1
If f(x) = 3x – 2I, P = \(\begin{pmatrix}2 & 1 \\-1 & 0 \end{pmatrix}\) and I is 2 x 2 identity matrix. Evaluate f(p)
- A. \(\begin{pmatrix}6 & -3 \\3 & 0 \end{pmatrix}\)
- B. \(\begin{pmatrix}2 & 0 \\0 & 2 \end{pmatrix}\)
- C. \(\begin{pmatrix}8 & 3 \\-3 & 2 \end{pmatrix}\)
- D. \(\begin{pmatrix}4 & 3 \\-3 & -2 \end{pmatrix}\)
Evaluate \(\begin{pmatrix}3 & -2 \\-7 & 5 \end{pmatrix}\) + 2\(\begin{pmatrix}-2 & 4\\3 & -1 \end{pmatrix}\)
- A. \(\begin{pmatrix}-1 & 6 \\-1 & 3 \end{pmatrix}\)
- B. \(\begin{pmatrix}3 & 4 \\-2 & 6 \end{pmatrix}\)
- C. \(\begin{pmatrix}-1 & 6 \\1 & 3 \end{pmatrix}\)
- D. \(\begin{pmatrix}3 & 4 \\2 & 6 \end{pmatrix}\)
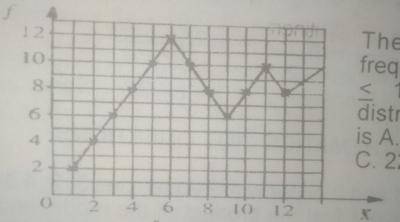
The cumulative frequency for 5\(\leq\) x \(\leq\) 12 from the distribution above is
- A. 22
- B. 40
- C. 46
- D. 72
The area of a square is 144 sqcm. Find the length of the diagonal.
- A. 13 cm
- B. 12√2 cm
- C. 12 cm
- D. 11√3 cm
Find the value of \(\frac{tan 60^o – tan 30^o}{tan 60^o + tan 30^o}\)
- A. \(\frac{4}{\sqrt{3}}\)
- B. \(\frac{2}{\sqrt{3}}\)
- C. 1
- D. \(\frac{1}{2}\)
Find the size of each exterior angle of a regular octagon
- A. 51o
- B. 45o
- C. 40o
- D. 36o
What is the value of k if the mid-point of the line joining (1 – k, – 4) and (2, k + 1) is (-k , k)?
- A. -3
- B. -1
- C. -4
- D. -2
Find the locus of point equidistant from two straight lines y – 5 = 0 and y – 3 = 0
- A. y - 2 = 0
- B. y - 4 = 0
- C. y - 1 = 0
- D. y - 3 = 0
A man 40 m from the foot of a tower observes the angle of elevation of the tower to be 30o. Determine the height of the tower.
- A. \(\frac{40\sqrt{3}}{3}m\)
- B. 20 m
- C. 40√3 m
- D. 40 m
If the lines 2y – kx + 2 = 0 and y + x – k/2 = 0 Intersect at (1, -2), find the value of k
- A. -4
- B. -3
- C. -2
- D. -1
A particle P moves between points S and T such that angles SPT is always constant of ST constant. Find the locus of P
- A. It is a semi circle with ST as diameter
- B. It is a perpendicular bisector of St
- C. It is a quadrant of a circle with ST as diameter
- D. It is a straight line perpendicular to ST
The volume of a hemispherical bowl is \(718\frac{2}{3}\). Find its radius .
- A. 4.0 cm
- B. 5.6 cm
- C. 7.0 cm
- D. 3.8 cm

In the parallelogram PQRS above, find angle SQR
- A. 100o
- B. 80o
- C. 50o
- D. 30o
If the lines 3y = 4x – 1 and qy = x + 3 are parallel to each other, the value of q is
- A. -4/3
- B. -3/4
- C. 4/3
- D. 3/4
Calculate the length of an arc of a circle diameter 14 cm, which substends an angle of 90o at the center of the circle
- A. \(\frac{7π}{2}\) cm
- B. 7π cm
- C. 14π cm
- D. \(\frac{7π}{4}\) cm
If X = {all the perfect squares less than 40}
Y = {all the odd numbers fro, 1 to 15}. Find X ∩ Y.
- A. {3, 9}
- B. {9}
- C. {9, 25}
- D. {1, 9}
Given
P = {1, 3, 5, 7, 9, 11}
and Q = {2, 4, 6, 8, 10, 12}. Determine the relationship between P and Q
- A. P∩Q = ∅
- B. P ⊂ Q
- C. Q⊂P
- D. P = Q
A man made a profit of 5% when he sold an article for N60,000.00. How much would he have sell the article to make a profit of 26%
- A. N68,000
- B. N72,000
- C. N65,000
- D. N70,000


