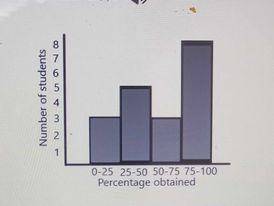
What is the general term of the sequence 3, 8, 13, 18, …?
- A. 5n - 2
- B. 5n + 2
- C. 5
- D. 5n
Solve the following quadratic inequality: \(x^2 – x\) – 4 β€ 2
- A. \(-3 < x < 2\)
- B. \(-2 β€ x β€ 3\)
- C. \(x β€ -2, x β€ 3\)
- D. \(-2 < x < 3\)
Let a binary operation ‘*’ be defined on a set A. The operation will be commutative if
- A. a*b = b*a
- B. (a*b)*c = a*(b*c)
- C. (b ΞΏ c)*a = (b*a) ΞΏ (c*a)
- D. None of the above
If \(-2x^3 + 6x^2 + 17x\) – 21 is divided by \((x + 1)\), then the remainder is
- A. 32
- B. 30
- C. -30
- D. -32
Find the matrix A
A \(\begin {bmatrix} 0 & 1\\2 & -1 \end {bmatrix}\) = \(\begin {bmatrix} 2 & -1\\1 & 0 \end {bmatrix}\)
- A. \(\begin {bmatrix} 2 & 1\\-^1/_2 & -^1/_2 \end {bmatrix}\)
- B. \(\begin {bmatrix} 0 & 1\\^1/_2 & ^1/_2 \end {bmatrix}\)
- C. \(\begin {bmatrix} 2 & 1\\0 & -1 \end {bmatrix}\)
- D. \(\begin {bmatrix} 2 & 1\\^1/_2 & -2 \end {bmatrix}\)
The ages of students in a small primary school were recorded in the table below.
| Age | 5-6 | 7-8 | 9-10 |
| Frequency | 29 | 40 | 38 |
Estimate the median.
- A. 7.725
- B. 6.225
- C. 7.5
- D. 6.5
A bag contains 8 red balls and some white balls. If the probability of drawing a white ball is half of the probability of drawing a red ball then find the probability of drawing a red ball and a white ball if the balls are drawn without replacement.
- A. \(\frac {1}{3}\)
- B. \(\frac {2}{9}\)
- C. \(\frac {2}{3}\)
- D. \(\frac {8}{33}\)
If A = { 1, 2, 3, 4, 5, 6}, B = { 2, 4, 6, 8 }. Find (A β B) β (B β A).
- A. {1, 3, 5, 8}
- B. {8}
- C. {1, 2, 3, 4, 5, 6, 8}
- D. {1, 3, 5}
Factorize: \(16x^4 – y^4\)
- A. \((2x - y)(2x + y)(4x^2 + y^2)\)
- B. \((2x + y)(2x + y)(4x^2 + y^2)\)
- C. \((2x - y)(2x - y)(4x^2 + y^2)\)
- D. \((2x - y)(2x + y)(4x^2 - y^2)\)
In a group of 500 people, 350 people can speak English, and 400 people can speak French. Find how many people can speak both languages.
- A. 750
- B. 850
- C. 250
- D. 150
Find the value of y, if log (y + 8) + log (y – 8) = 2log 3 + 2log 5
- A. y = Β±5
- B. y = Β±10
- C. y = Β±17
- D. y = Β±13
Determine the area of the region bounded by y = \(2x^2\) + 10 and Y = \(4x + 16\).
- A. 18
- B. \(\frac {-10}{3}\)
- C. \(\frac {44}{3}\)
- D. \(\frac {64}{3}\)
Calculate the mean deviation of the first five prime numbers.
- A. 2.72
- B. 5.6
- C. 5.25
- D. 13.6
Differentiate the function y = \(\sqrt[3]{x^2}(2x – x^2)\)
- A. \(\frac {dy}{dx} = \frac {10x^{5/3}}{3} - \fracΒ {8x^{2/3}}{3}\)
- B. \(\frac {dy}{dx} = \frac {10x^{2/3}}{3} - \fracΒ {8x^{5/3}}{3}\)
- C. \(\frac {dy}{dx} = \frac {10x^{5/3}}{3} - \frac {8x^{5/3}}{3}\)
- D. \(\frac {dy}{dx} = \frac {10x^{2/3}}{3} - \frac {8x^{2/3}}{3}\)
Find the equation of straight line passing through (2, 3) and perpendicular to the line \(3x + 2y + 4 = 0\)
- A. 3y = 5x - 2
- B. y = \(\frac {5}{3} \times - 2\)
- C. None of these
- D. 3y = 2x + 5
A coin is thrown 3 times. What is the probability that atleast one head is obtained?
- A. \(\frac {7}{8}\)
- B. \(\frac {3}{8}\)
- C. None the above
- D. \(\frac {1}{8}\)
A boat sails 8 km north from P to Q and then sails 6 km west from Q to R. Calculate the bearing of R from P. Give your answer to the nearest degree.
- A. 217\(^o\)
- B. 323\(^o\)
- C. 037\(^o\)
- D. 053\(^o\)
If D = \(\begin{bmatrix}2& -1&3\\4&1&2\\1&-3&1\\\end{bmatrix}\)
Find |D|
- A. 16
- B. 14
- C. -23
- D. -37
A committee of 5 people is to be chosen from a group of 6 men and 4 women. How many committees are possible if there is to be a majority of women?
- A. 60
- B. 15
- C. 66
- D. 4