Find the area and perimeter of a square whose length of diagonals is 20\(\sqrt2\) cm.

Explanation

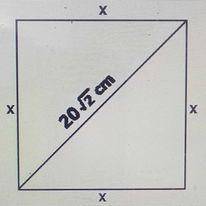
Using Pythagoras theorem

⇒ \((20\sqrt2)2 = x^2 + x^2\)

⇒ 800 = \(2x^2\)

⇒ 400 = \(x^2\)

⇒ x = \(\sqrt400\) = 20 cm

∴ Area of a square = \(x^2 = 20^2 = 400 cm^2\)

∴ Perimeter of a square = 4x = 4 x 20 = 80 cm

There is an explanation video available .