a. A car travels a distance of 112 km at an average speed of 70 km/h. it then travels a further 60 km at an average speed of 50 km/h. Calculate, for the entire journey, the total time taken.
b. if \(\frac{x}{y}\) = 2 and \(\frac{y}{z}\) = 3, find the value of \(\frac{ x + y}{y + z }\)
Find the mean deviation of assets of numbers: 14, 15, 16, 17, 18, and 19.
- A. 2.5
- B. 1.7
- C. 1.5
- D. 3.5
M varies jointly as the square of n and square root of q. If M = 24 when n = 2 and q = 4, find M when n = 5, q = 9.
- A. 288
- B. 400
- C. 300
- D. 225
The diagonals of a rhombus are 16 cm and 12 cm find the length of the side.
- A. 20cm
- B. 8cm
- C. 14cm
- D. 10cm
If 2x – 3y = -11 and 3x + 2y = 3, evaluate \( (y – x)^2\)
- A. 16
- B. 25
- C. 9
- D. 4
A notebook of length 15 cm was measured to be 16.8 cm, calculate, correct to two d.p, the percentage error in the measurement.
- A. 12.00%
- B. 11.71%
- C. 10.71%
- D. 11.21%
Find the gradient of the line passing through the points \((\frac{1}{2}, \frac{- 1}{3}) and ( 3 , \frac{2}{3})\)
- A. \(\frac{2}{5}\)
- B. \(\frac{5}{2}\)
- C. \(\frac{2}{7}\)
- D. \(\frac{7}{2}\)

Find the value of m in the diagram above.
- A. \(40^0\)
- B. \(50^0\)
- C. \(130^0\)
- D. \(140^0\)

In the diagram above, O is the centre of a circle NST. |NT| = |ST| and ∠NTS = 36°. Find the measure of the angle marked t.
- A. \(72^0\)
- B. \(54^0\)
- C. \(36^0\)
- D. \(108^0\)
The length of the diagonal of a square is 12 cm. Calculate the area of the square.
- A. \(36 cm^2\)
- B. \(48 cm^2\)
- C. \(72 cm^2\)
- D. \(18 cm^2\)
Find the quadratic equation whose roots are \(\frac{2}{3} and \frac{- 3}{4}\)
- A. \(12y^2 - y - 6 = 0\)
- B. \(12y^2 - y + 6 = 0\)
- C. \(12y^2 + y - 6 = 0\)
- D. \(y^2 + y - 6 = 0\)

In the diagram, NR is a diameter, ∠MNR = x° and, ∠SRN = (5x + 20)°. Find the value of 2x.
- A. \(42^0\)
- B. \(35^0\)
- C. \(20^0\)
- D. \(90^0\)
The price of a shoe was decreased by 22%. If the new price is $27.3. what is the original price.
- A. $62.30
- B. $42.30
- C. $72.00
- D. $35.00
Write the name of a triangle with the vertices (1, -3), (6, 2) and (0,4)?
- A. Scalene triangle
- B. Isosceles triangle
- C. Right -angle triangle
- D. Equilateral triangle
Consider the statements:
p: Siah is from Foya.
q: Foya is in Lofa.
Write in symbolic for the statement: “If Siah is from Foya, then Foya is in Lofa”
- A. ~q ⇔ p
- B. q ⇒ p
- C. p ⇒ q
- D. p ⇔ q
Mrs Kebeh stands at a distance of 110 m away from a building of vertical height 58 m. If Kebeh is 2 m tall, find the angle of elevation of the top of the building from her eye.
- A. \(27^0\)
- B. \(28^0\)
- C. \(20^0\)
- D. \(26^0\)
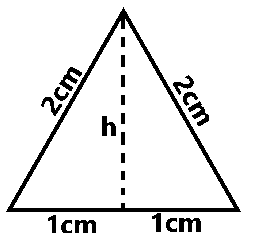
An equilateral triangle has a side 2 cm. Calculate the height of the triangle.
- A. 5cm
- B. \(\sqrt5\)cm
- C. 3cm
- D. \(\sqrt{ 3}\)cm
Factorize completely: \(x^2 – (y + z)^2\)
- A. (x - y - z)(x - y - z)
- B. (x + y + z)(x - y - z)
- C. (x + y + z)(x + y - z)
- D. (x + y - z)(x - y + z)
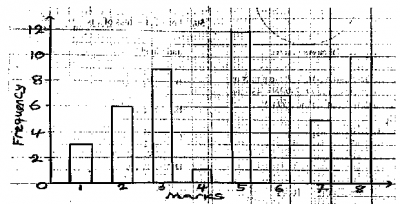
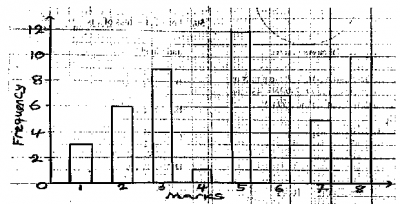
What percentage of the students scored at most 5 marks?
- A. 58.5%
- B. 63.2%
- C. 38.3%
- D. 41.5%