(a) Copy and complete the following table of values for \(y = 2x^{2} – 9x – 1\).
| x | -1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| y | -1 | -8 | -11 | 17 |
(b) Using a scale of 2cm to represent 1 unit on the x- axis and 2cm to represent 5 units on the y- axis, draw the graph of \(y = 2x^{2} – 9x – 1\).
(c) Use your graph to find the : (i) roots of the equation \(2x^{2} – 9x = 4\), correct to one decimal place ; (ii) gradient of the curve \(y = 2x^{2} – 9x – 1\) at x = 3.
Explanation
(a)
| x | -1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| y | 10 | -1 | -8 | -11 | -10 | -5 | 4 | 17 |
(b) 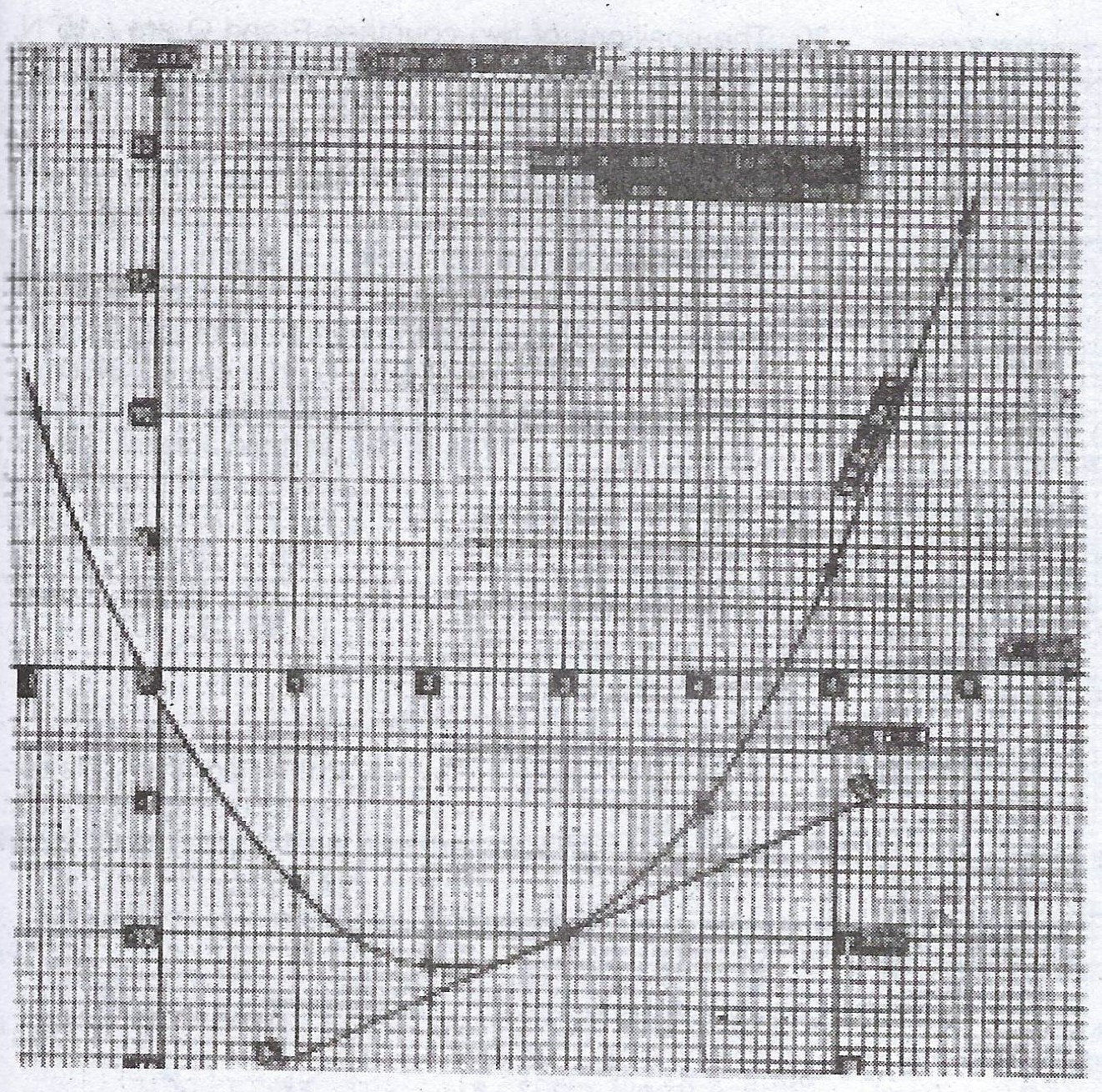
(c)(i) \(2x^{2} - 9x = 4\)
\(2x^{2} - 9x - 1 = 4 - 1 = 3\)
At y = 3, x = 0.2 and 4.3.
(ii) Gradient of \(y = 2x^{2} - 9x - 1\) at x = 3
= \(\frac{BC}{AB} = \frac{9.5}{4.3}\)
= \(2.2\)

