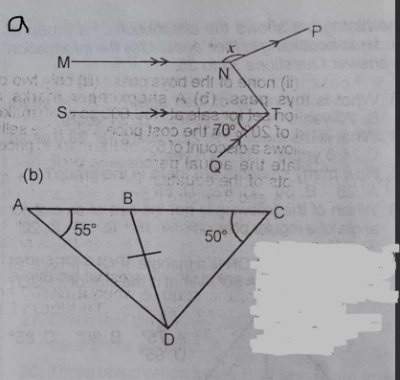
(a) see figure a above, In the diagram, MN || ST, NP || QT and < STQ = 70°. Find x.
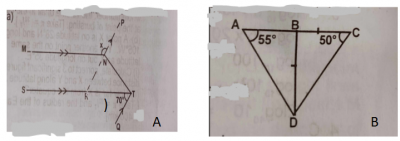
(b) see figure b above, In the diagram above, AC is a straight line, |BC| = |BD|, \(\stackrel\frown{BCD} = 50°\) and \(\stackrel\frown{BAD} = 55°\). Find \(\stackrel\frown{BDA}\).
Explanation
(a) see figure above a \(< QTR = < TRP = 70°\) (alternate angle)
\(< MNR = < TRP = 70°\) (alternate angle; MN || ST)
\(< MNR + < PNM = 180°\)
\(70° + < PNM = 180° \implies < PNM = 110°\)
\(\therefore x = 110°\)
(b)see figure b above \(< BDC = < BCD = 50°\) (BD = BC)
\(\therefore \Delta BDC = isosceles\)
\(< ABD = 2(50°) = 100°\) (exterior angle)
\(55° + < BDA + 100° = 180°\)
\(< BDA = 180° - 155° = 25°\)

