The following data gives the lengths, in cm, of 30 pieces of iron rods :
45 55 65 60 61 68 59 54 64 76 50 68 72 68 80 67 70 62 79 67 64 63 71 59 64 53 57 74 55 57
(a) Using class intervals of 45 – 49, 50 – 54, 55 – 59, … construct a frequency table of the data.
(b) Draw the histogram for the distribution
(c) Calculate the mean of the distribution
(d) What is the probability of selecting an iron rod whose length is in the modal class?
Explanation
(a)
|
Class Interval |
Freq (f) | Tally |
Mid-point (x) |
\(fx\) |
| 45 - 49 | 1 | | | 47 | 47 |
| 50 - 54 | 3 | ||| | 52 | 156 |
| 55 - 59 | 6 | 57 | 342 | |
| 60 - 64 | 7 | 62 | 434 | |
| 65 - 69 | 6 | 67 | 402 | |
| 70 - 74 | 4 | |||| | 72 | 288 |
| 75 - 79 | 2 | || | 77 | 154 |
| 80 - 84 | 1 | | | 82 | 82 |
| \(\sum\) | 30 | 1905 |
(b) 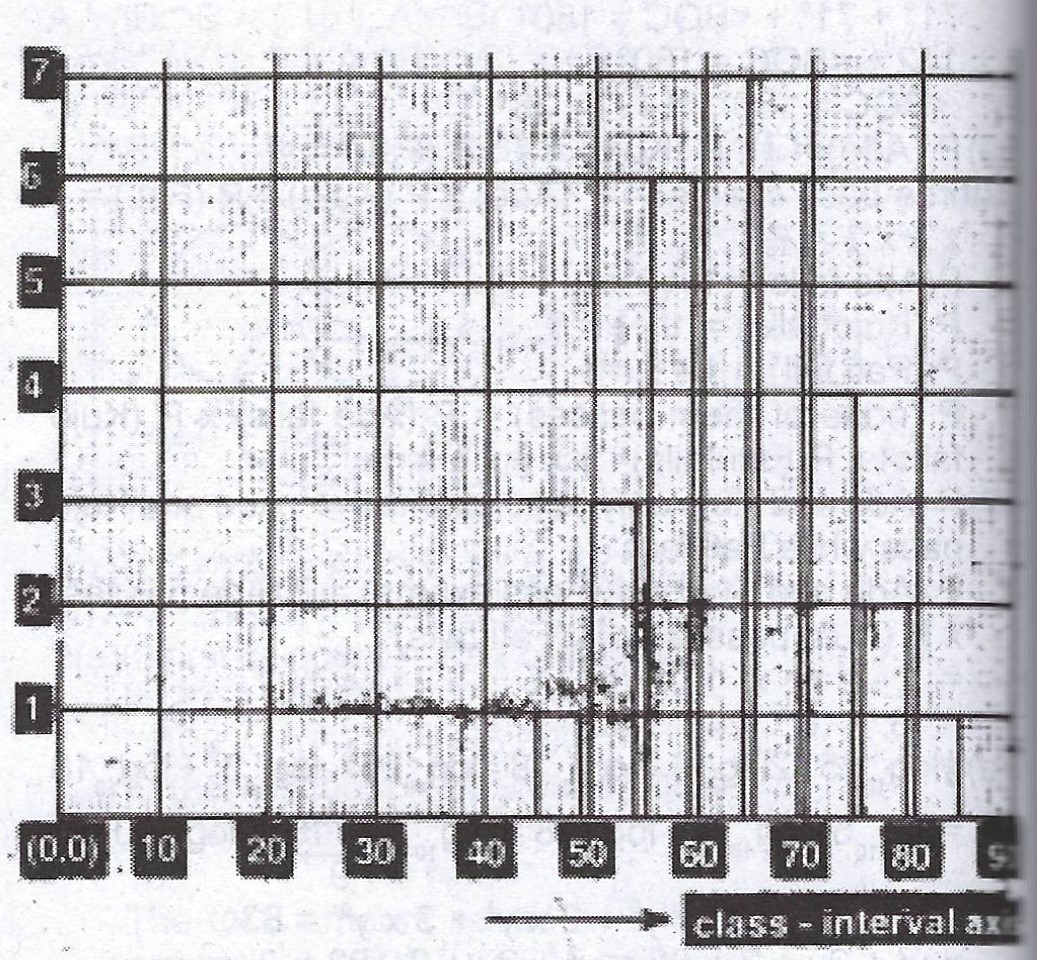
(c) Mean \(\bar{x} = \frac{\sum fx}{\sum f}\)
= \(\frac{1905}{30}\)
= \(63.5\).
(d) P(iron rod in modal class) = \(\frac{7}{30}\)

