(a) If \(x = \begin{pmatrix} 2 \\ 3 \end{pmatrix}, y = \begin{pmatrix} 5 \\ -2 \end{pmatrix}\) and \(z = \begin{pmatrix} -4 \\ 13 \end{pmatrix}\), find the scalars p and q such that \(px + qy = z\).
(b)(i) Using the scale of 2cm to 2 units on both axis, draw on a graph paper two perpendicular axis x and y for \(-5 \leq x \leq 5, -5 \leq y \leq 5\) respectively.
(ii) Draw, on the graph paper, indicating clearly the vertices and their coordinates,
(1) the quadrilateral WXYZ with W(2, 3), X(4, -1), Y(-3, -4) and Z(-3, 2).
(2) the image \(W_{1}X_{1}Y_{1}Z_{1}\) of the quadrilateral WXYZ under an anti-clockwise rotation of 90° about the origin where \(W \to W_{1}, X \to X_{1}, Y \to Y_{1}\) and \(Z \to Z_{1}\).
Explanation
(a) px + qy = z
\(p \begin{pmatrix} 2 \\ 3 \end{pmatrix} + q \begin{pmatrix} 5 \\ -2 \end{pmatrix} = \begin{pmatrix} -4 \\ 13 \end{pmatrix}\)
\(\begin{pmatrix} 2p + 5q \\ 3p - 2q \end{pmatrix} = \begin{pmatrix} -4 \\ 13 \end{pmatrix}\)
Thus,
\(2p + 5q = -4 .....(1)\)
\(3p - 2q = 13 ......(2)\)
Solving the equations, we have p = 3 and q = -2.
(b)(i) 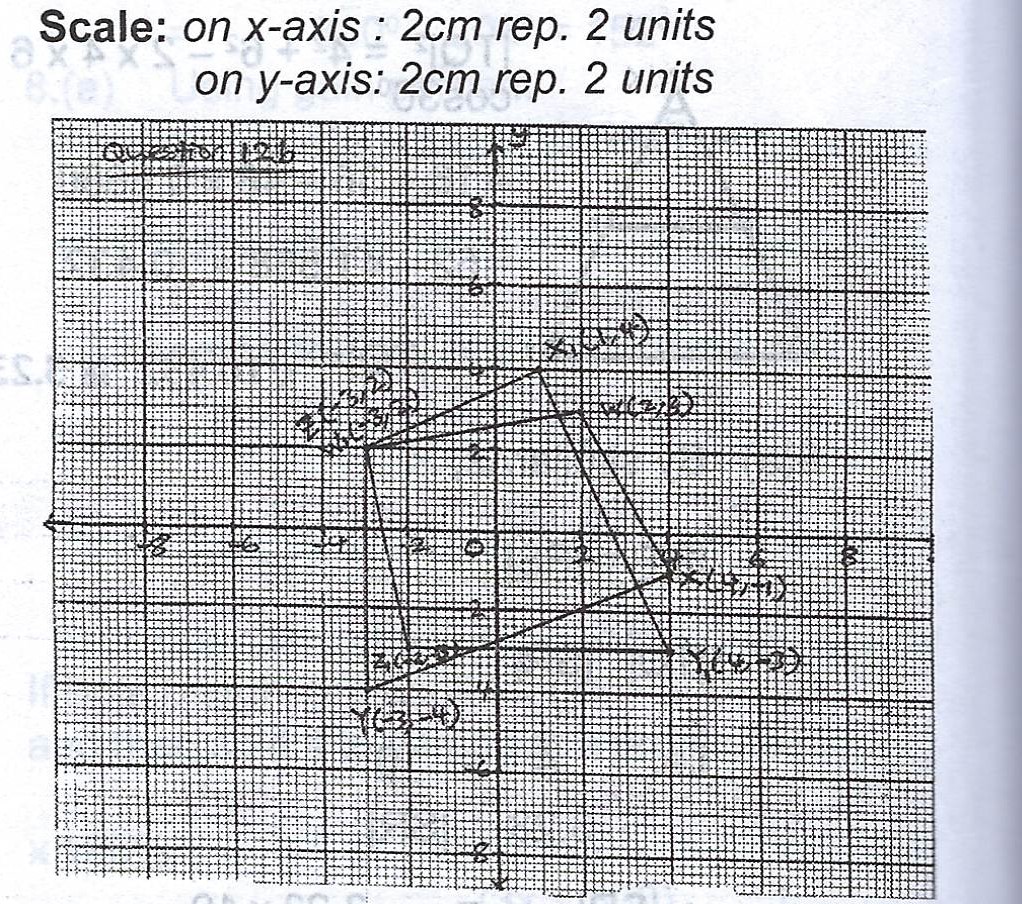
(ii) See attached graph for the quadrilateral W(2, 3), X(4, -1), Y(-3, -4) and Z(-3,2).
(iii) See attached for the image for the quadrilateral WXYZ under an anticlockwise rotation of 90° is \(W_{1}(-3, 2), X_{1}(1, 4), Y_{1}(4, -3)\) and \(Z_{1}(-2, -3)\).

