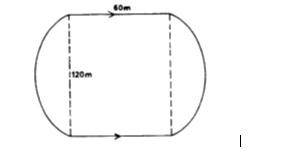
The diagram shows an athletics track with two parallel sides and two semi-circular ends Each of the parallel sides is 60 metres, long and the diameter of each semi-circular end is 120 metres long.
(a) Calculate the distance covered by an athlete who runs round the tack the two times. [Take \(\pi\) = \(\frac{22}{7}\)]
(b) If the athlete spends 200 seconds for the race, calculate the speed in km/h.
Explanation
The Chief Examiner reported that quite a handful of the Candidates' got part (a) correctly but couldn't establish the relationship between km/h and m/s.
(a) The distance covered = (2 x 60) + (2 x \(\frac{22}{7}\)) = 120 + 377.142 = 497.1429m so that if the athlete runs two times will covered = 2 x 497.1429
m = 994.2857m (at least one decimal place).
(b) The speed m/s = \(\frac{994,2857}{200}\) and converting to km/h we will have \(\frac{994,2857}{1000} \times \frac{200}{3600}\) = 17.8973 km/h

