ANWSER
—
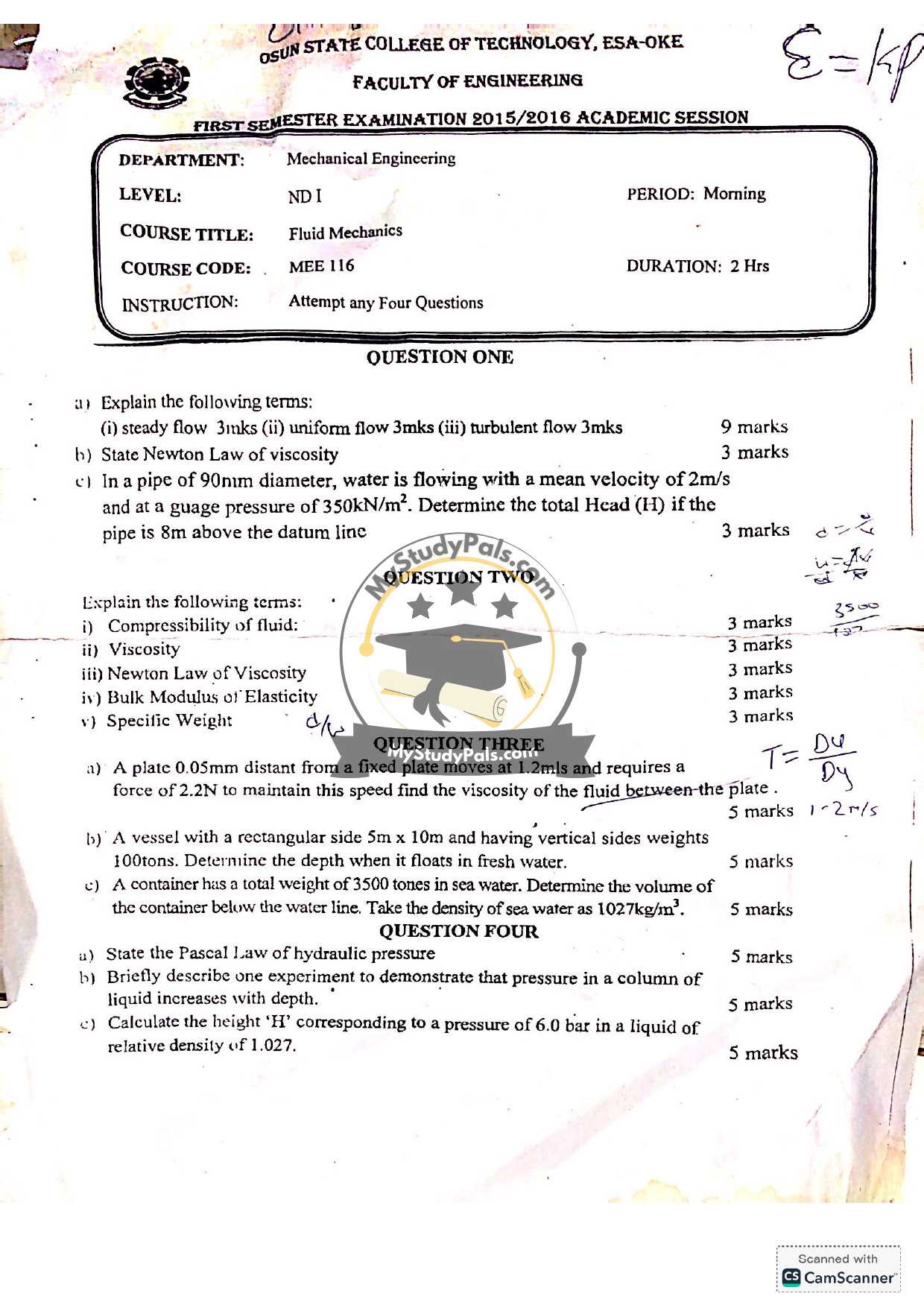
Question 1:
a)
(i) Steady flow: A flow where the fluid’s velocity, pressure, and other properties at any point do not change with time.
(ii) Uniform flow: A flow where the fluid’s velocity and other properties are the same at every point in the flow field at any given time.
(iii) Turbulent flow: A flow characterized by chaotic, irregular motion of fluid particles, with rapid mixing and fluctuations in velocity and pressure.
b) Newton’s Law of Viscosity: The shear stress (\( \tau \)) between adjacent fluid layers is proportional to the velocity gradient (\( \frac{du}{dy} \)) perpendicular to the flow direction, i.e., \( \tau = \mu \frac{du}{dy} \), where \( \mu \) is the dynamic viscosity.
c)
Given: Diameter \( D = 90 \, \text{mm} = 0.09 \, \text{m} \), Velocity \( V = 2 \, \text{m/s} \), Pressure \( P = 350 \, \text{kN/m}^2 \), Datum height \( z = 8 \, \text{m} \).
Total Head \( H \) is given by:
\[ H = \frac{P}{\rho g} + \frac{V^2}{2g} + z \]
Assume \( \rho = 1000 \, \text{kg/m}^3 \) and \( g = 9.81 \, \text{m/s}^2 \).
\[ H = \frac{350 \times 10^3}{1000 \times 9.81} + \frac{2^2}{2 \times 9.81} + 8 \]
\[ H = 35.68 + 0.20 + 8 = 43.88 \, \text{m} \]
—
Question 2:
i) Compressibility of fluid: The measure of how much a fluid’s volume decreases under pressure.
ii) Viscosity: A fluid’s resistance to shear or flow, caused by internal friction between fluid layers.
iii) Newton’s Law of Viscosity: As stated in Question 1(b).
iv) Bulk Modulus of Elasticity: The ratio of compressive stress to volumetric strain, indicating a fluid’s resistance to compression.
v) Specific Weight: The weight per unit volume of a fluid, \( \gamma = \rho g \).
—
Question 3:
a) Given: Distance \( Dy = 0.05 \, \text{mm} = 0.00005 \, \text{m} \), Velocity \( V = 1.2 \, \text{m/s} \), Force \( F = 2.2 \, \text{N} \).
Shear stress \( \tau = \frac{F}{A} \). Assume plate area \( A \) is not given; likely \( \tau = \frac{2.2}{A} \).
Using \( \tau = \mu \frac{dV}{dy} \):
\[ \mu = \frac{\tau \cdot Dy}{V} = \frac{2.2 \times 0.00005}{1.2 \times A} \]
If \( A \) is \( 1 \, \text{m}^2 \), \( \mu = 9.17 \times 10^{-5} \, \text{Pa·s} \).
b) Given: Weight \( W = 100 \, \text{tons} = 100 \times 10^3 \, \text{kg} \), Area \( A = 5 \times 10 = 50 \, \text{m}^2 \).
Buoyant force \( F_b = W \).
\[ \rho g V = W \implies V = \frac{W}{\rho g} = \frac{100 \times 10^3}{1000 \times 9.81} = 10.19 \, \text{m}^3 \]
Depth \( d = \frac{V}{A} = \frac{10.19}{50} = 0.20 \, \text{m} \).
c) Given: Weight \( W = 3500 \, \text{tons} = 3500 \times 10^3 \, \text{kg} \), Density \( \rho = 1027 \, \text{kg/m}^3 \).
Volume \( V = \frac{W}{\rho} = \frac{3500 \times 10^3}{1027} = 3408 \, \text{m}^3 \).
—
Question 4:
a) Pascal’s Law: Pressure applied to a confined fluid is transmitted undiminished in all directions and acts equally on all surfaces.
b) Experiment: A tall container with holes at different depths filled with water. Water jets from lower holes travel farther, showing higher pressure with depth.
c) Given: Pressure \( P = 6.0 \, \text{bar} = 6 \times 10^5 \, \text{Pa} \), Relative density \( RD = 1.027 \).
Density \( \rho = 1.027 \times 1000 = 1027 \, \text{kg/m}^3 \).
\[ P = \rho g H \implies H = \frac{P}{\rho g} = \frac{6 \times 10^5}{1027 \times 9.81} = 59.5 \, \text{m} \].
—
Question 5:
a) Archimedes’ Principle: A body immersed in a fluid experiences an upward buoyant force equal to the weight of the displaced fluid.
b)
(i) Buoyancy: Upward force due to displaced fluid.
(ii) Metacenter: Intersection point of buoyant force and body’s axis when tilted.
(iii) Metacentric height: Distance between metacenter and center of gravity, determining stability.
c) Hydrometer:
(i) Description: A weighted glass tube with a graduated stem.
(ii) Working principle: Floats at a level depending on fluid density.
(iii) Uses: Measures liquid density (e.g., battery acid, alcohol content).
—
Question 6:
a) Bernoulli Equation: \( P_1 + \frac{1}{2} \rho V_1^2 + \rho g z_1 = P_2 + \frac{1}{2} \rho V_2^2 + \rho g z_2 \).
b) Energy equation: Total energy = Pressure energy + Kinetic energy + Potential energy.
c) Given: \( D_1 = 300 \, \text{mm} \), \( D_2 = 150 \, \text{mm} \), \( Q = 40 \, \text{l/s} = 0.04 \, \text{m}^3/\text{s} \), \( z_1 = 10 \, \text{m} \), \( z_2 = 6 \, \text{m} \), \( P_1 = 400 \, \text{kN/m}^2 \).
Velocities:
\[ V_1 = \frac{Q}{A_1} = \frac{0.04}{\pi (0.15)^2} = 0.57 \, \text{m/s} \]
\[ V_2 = \frac{Q}{A_2} = \frac{0.04}{\pi (0.075)^2} = 2.26 \, \text{m/s} \]
Using Bernoulli:
\[ P_2 = P_1 + \frac{1}{2} \rho (V_1^2 – V_2^2) + \rho g (z_1 – z_2) \]
\[ P_2 = 400 \times 10^3 + 500 (0.57^2 – 2.26^2) + 1000 \times 9.81 \times 4 \]
\[ P_2 = 400 \times 10^3 – 2.42 \times 10^3 + 39.24 \times 10^3 = 436.82 \, \text{kN/m}^2 \].
—
Question 7:
a) Fluid mechanics: Study of fluids (liquids/gases) at rest or in motion, and their interactions.
b) Fluid: A substance that deforms continuously under shear stress, including liquids and gases.
c) Given: \( W_{\text{earth}} = 21 \, \text{kgf} \), \( W_{\text{loc}} = 7 \, \text{kgf} \).
\[ g_{\text{loc}} = g_{\text{earth}} \times \frac{W_{\text{loc}}}{W_{\text{earth}}} = 9.81 \times \frac{7}{21} = 3.27 \, \text{m/s}^2 \].
d) Given: \( m = 900 \, \text{kg} \), \( F = 1650 \, \text{N} \).
\[ a = \frac{F}{m} = \frac{1650}{900} = 1.83 \, \text{m/s}^2 \].
e) Given: Empty bottle \( W = 0.027 \, \text{kg} \), Water \( W = 0.052 \, \text{kg} \), Liquid \( W = 0.367 \, \text{kg} \).
Mass of water \( = 0.052 – 0.027 = 0.025 \, \text{kg} \).
Mass of liquid \( = 0.367 – 0.027 = 0.340 \, \text{kg} \).
Density \( \rho_{\text{liquid}} = \frac{0.340}{0.025} \times 1000 = 13600 \, \text{kg/m}^3 \). Likely mercury.
—